A PROBABILISTIC APPROACH TO RISKS TO INTRUDERS AND
NEARBY POTENTIALLY EXPOSED GROUPS
Gary B. Merrell and Arthur A. Sutherland
Rogers & Associates Engineering Corporation
Robert E. Donders
Atomic Energy of Canada Ltd.
ABSTRACT
In some analyses of the long-term impacts of low-level radioactive waste disposal facilities potential radiation doses and risks to intruders can have an important influence on the kinds of waste that can be emplaced. Many of the estimates of impacts on intruders are based on the assumption that after a certain period after disposal facility closure, usually 100 years, intruders are free to invade the disposal facility site and conduct almost any activity on the site that is possible. The remediation of existing contaminated areas often involves removing low-level waste and placing it in disposal cells that will be watched more or less in perpetuity. In those cases at least, intruders are not likely to have free reign at the site for much longer than 100 years, if ever.
This paper explores the effects on the maximum potential doses to intruders of assigning probabilities to several conditions that would have to exist before an intruder could receive a significant impact from the waste in a disposal facility. It shows that even assigning conservatively high probabilities that those conditions occur could result in allowing disposal of wastes that have higher concentrations of radioactive contaminants or less restrictive waste forms.
INTRODUCTION
The latest U.S. Nuclear Regulatory Commission (NRC) draft Branch Technical Position on a Performance Assessment Methodology for Low-Level Radioactive Waste Disposal Facilities (Ref. 1) specifically recommends not assigning probabilities to various scenarios of exposure to contaminants from low-level radioactive waste (LLW) disposal facilities. This can have major impact on the estimation of potential doses to intruders at the disposal site once institutional control is assumed to be lost. This, coupled with the 100-years-only approach to institutional control, can result in projections of high risks and doses that result from intruder exposure scenarios starting immediately after the 100 year period ends.
Some agencies, such as the U.S. Department of Energy (DOE), are interested in establishing longer periods of control over sites for disposal of LLW from cleanup activities, sometimes approaching a situation similar to perpetual control over existing DOE sites. There is clearly a conflict between assuming that anything can happen at a site once 100 years has passed since closure and the desire to take credit for control over uses of the site for longer periods of time, even if the possibility of accidental loss of control after 100 years is recognized. This conflict will be debated in various regulatory and public forums more intensely as remediation efforts lead to increasing disposal of LLW from remedial activities in facilities dedicated to that purpose. In recognition of the fact that it may be necessary to bar the public from sizable areas of cold war weapons facilities indefinitely, this paper addresses employing probabilities in estimating the occupation and use of locations near, but not coincident, with the actual disposal facility, as well as those directly over the waste.
Time-varying probabilities could be assigned to such key items as: The failure of active and passive institutional controls, failure of engineered features that protect the intruder, occupation of the site, use of contaminated well water, and growing food on site in contaminated soil. The time-varying probabilities for any of these factors, and possibly others, in the estimation of risks and doses are to be proposed by the risk assessors and must then be approved by the regulators. Time-varying intruder probabilities have been considered in the preliminary safety analysis for the proposed Intrusion-Resistant Underground Structure disposal facility in Canada (Ref. 2).
This paper discusses a probabilistic approach to intruder scenarios and demonstrates the difference between projected intruder doses using a probabilistic approach and the one usually used in the United States. It presents an approach to intruder scenarios that represents a middle ground between the two ways of treating doses and risks to intruders described above.
PROBABILITIES ASSOCIATED WITH INTRUSION SCENARIOS
At any time during the period covered by a long-term performance assessment there is a possibility that individuals may occupy a nearby restricted area or the actual disposal facility site. Such events must be preceded by the failure of certain protective measures designed to prevent individuals from occupying the site or its surrounding area, and being exposed to contaminants in the waste. The protective measures are generally the active and passive institutional controls and the engineered features of the facility. In addition to these three protective measures, an intrusion event must be preceded by an individual's intent to move into the restricted area. Probabilities can be assigned for the individual's intent to occupy the site and for the failure of the protective measures. This section briefly describes six parts of intrusion scenarios that could be treated probabilistically.
After an intrusion event occurs, additional probabilities can be assigned to certain of the intruder's activities and potential exposures on or near the site. For example, an intruder may or may not use contaminated groundwater from beneath the site. Also, the intruder locating on top of the disposed waste may or may not grow food there. Examples of these probabilities and the intrusion probabilities mentioned above are discussed in the section on example probabilities.
The probability of an intrusion event occurring can be estimated by combining the probabilities of a series of steps leading up to the intrusion. Up to six probability factors could be combined to estimate the likelihood of an individual occupying the disposal facility site or its vicinity, and being exposed to contaminants from the waste. The six factors are:
An example application of the above six probability factors is described below. The example is representative of a near-surface disposal facility in a humid climate with the following characteristics:
EXAMPLE PROBABILITIES
Intent to Occupy the Site Area (P1)
Before an individual resides on or near the disposal facility site, there must first be an intent to do so. This can be the intent of an individual to build a house on the site or the intent of a land developer to build a number of houses. The probability of intent to occupy the site could be calculated based on the product of the population density and the site area divided by the average number of persons per household. For the example here the probability was conservatively set equal to one after year 100, which could apply to relatively populous regions of North America. Prior to year 100, the site will be controlled and the probability of intent to occupy the site is assumed to be zero.
Failure of Active Institutional Controls (P2)
Before and individual can reside on or near the disposal facility site, there must be a failure of active institutional controls at the site. Assuming that the site will be fenced and guarded for at least the first 100 years after closure, the probability of failure is set equal to zero for the first 100 years. After year 100, control of the site is less certain and the benefits of active institutional control are phased out in this example by assuming that the failure probability increases linearly from zero to one between years 100 and 200. After year 200 no credit is taken for active institutional control and P2 remains at a constant value of one for the rest of the analysis.
Failure of Passive Institutional Controls (P3)
In addition to active control of the area surrounding the disposal facility after the facility is closed, several passive control measures are assumed to exist. These include local land deed restrictions, federal and state government records, and markers on the site. For this example the probability that these passive measures will fail to deter intruders is assumed to depend on the particular scenario. Different probabilities are used for individuals who occupy the area over the waste (intruders, referred to as "onsite" persons), and persons who occupy the supposedly restricted areas nearby (referred to as "offsite") and use groundwater and stream water.
The onsite intruder scenario has the lowest probability of occurrence because of the markers on the waste site. The probability that these passive institutional controls will fail is assumed to increase linearly from zero to one during the period from year 100 to year 1,000. The probability is designated P3OS (for onsite). After year 1,000, no further credit is taken for the passive controls on the site, so P3OS remains at a value of one.
For the offsite groundwater scenario, the passive institutional controls are assumed to remain partially effective for up to 300 years. The probability of failure, designated as P3DGP (for downgradient plume), is assumed to increase linearly from zero to one during years 100 to 300. After year 300, no further credit is taken and the probability remains at a value of one.
The offsite stream water scenario is the least likely to be prevented by passive institutional controls. The probability of failure, designated as P3DGS (for downgradient stream), is assumed to equal one for all times after year 100.
Failure of Engineered Features to Prevent Intrusion (P4)
The disposal facility design may include some material in the earthen cover system that is intended to serve as a warning barrier to anyone digging into the cover. While the barrier is not expected to be completely effective at preventing intrusion into the waste, it may serve to convey a message to future individuals that the site was designed or engineered for a specific purpose. As such, it may provide a deterrent to future onsite intrusion because it would warn away the intruder. For purposes of this example, P4 was given a value of 0.5, indicating that the intruder warning layer would always have a 0.5 probability of preventing intrusion into the waste by an individual who digs into the cover system.
Obviously, the probability of engineered feature failure would only affect an intruder who digs into the cover over the waste; it would not apply to the offsite groundwater or stream water scenarios. Therefore, P4 was not used in calculating probabilities for the offsite scenarios.
Probability of Gardening (P5)
The above probabilities P1, P2, P3OS, and P4, when multiplied together, give the probability that an intruder will occupy the land over the waste and disturb the waste. There is the possibility that, if an intruder does reside over the waste, he or she will grow food. Given that the onsite intrusion occurs, the conditional probability that the intruder will also grow a garden in contaminated soil is assumed to be 0.2 for the entire period of interest. Thus, P5 is set equal to 0.2 for the onsite scenarios and is not used for the offsite scenarios.
Probability of Using Well Water (P6)
Given that an intrusion occurs, the individual may or may not use groundwater from an aquifer contaminated by releases from the disposed waste. The individual may use a municipal water supply system or uncontaminated well water (e.g., from another aquifer). In light of these possibilities, the probability of using the contaminated well water was assumed to be 0.2. Thus, P6 has a constant value of 0.2.
The probability P6 could be further divided into two separate probabilities, depending on whether the onsite intruder only uses the well water for culinary purposes or also uses it to water a garden. That division is not made here; rather, it is assumed that the onsite intruder always uses the contaminated well water both for culinary purposes and to irrigate a garden.
CALCULATING PROBABILITIES OF EXPOSURE
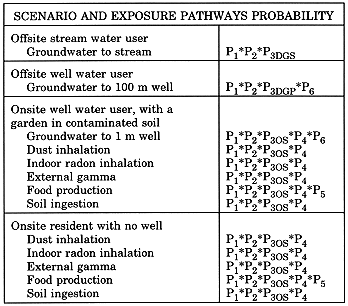
The probabilities P1 through P6 described above were used to calculate probabilities for a range of intruder exposures. Those exposures, in turn, represent sets of pathways. For the onsite scenarios, the pathways include well water consumption, contaminated dust inhalation, indoor radon inhalation, exposure to external gamma radiation, the production of food in contaminated soil, and contaminated soil ingestion from dirty hands and produce. In addition to the onsite intruder scenarios, probabilities of exposures were calculated for users of nearby well water and stream water who are not directly over the waste. Four scenarios were evaluated:
Each scenario and exposure pathway has associated with it a probability of occurrence. The probable dose for each of the four scenarios was calculated by multiplying the pathway-specific doses by the appropriate probabilities. Table I shows the scenarios, exposure pathways, and probabilities used to calculate the risks.
Table I. Scenario and Pathway Probabilities

When calculating probable doses for each of the four scenarios in Table I, the calculated probable doses (i.e., the products of doses and related probabilities) would be summed over the exposure pathways to obtain the total scenario probable dose. Since both the doses and the probabilities vary with time, the probable doses will also be time dependent. The probability component of the probable doses always increases with time while the doses can increase or decrease with time, depending on the particular pathway. For example, dust inhalation and gamma doses generally decrease with time due to radioactive decay of the contaminants. In contrast, indoor radon doses may increase with time due to the ingrowth of radium-226. Annual doses from most radionuclides that move through water pathways will rise to a peak value at some time and then decline, depending on their mobility, release rates, and half-lives.
An example of a time-varying probability for the onsite intruder scenario with use of contaminated well water is given in Fig. 1. The figure represents the time history of the product of P1, P2, P3OS, P4, and P6.
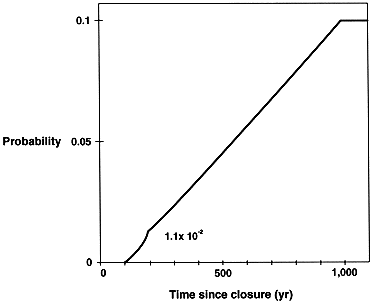
Figure 1.Growth of Probabilities for the Onsite Intruder Using Contaminated Well Water
In Fig. 1 the probability of this intrusion scenario increases as the square of time between 100 and 200 years after closure because the probabilities of failure of both active and passive institutional control increase linearly over that period. The probability of the residential intruder scenario grows linearly with time from 200 to 1,000 years, at which time the probability of failure of passive institutional control reaches one. A constant probability of less than one continues indefinitely, based on the assumption that features of the cover over the waste will always discourage intruders.
Figure 2 is a scale plot of the probabilities of the four scenarios. Of course the scenario probabilities shown are entirely dependent on the choices of individual probabilities described in the section on example probabilities. Different choices of individual probabilities will cause different scenario probabilities to result.
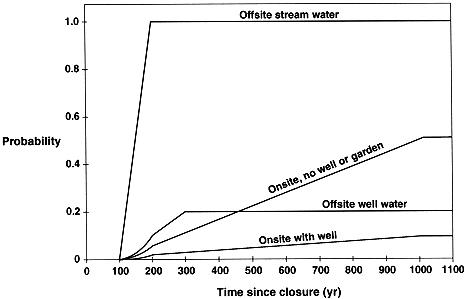
Figure 2. Dose Reduction for Example Scenarios
The lower curve in Fig. 2 is the same history of probability given in Fig. 1. All three of the other scenarios have higher probabilities of occurrence than the onsite resident who uses a well and grows a garden in contaminated soil. The probability of a member of the public entering the restricted area and using water from a stream that might be contaminated by releases from the disposal facility (the offsite stream water user scenario) reaches one 200 years after facility closure. The probability of a member of the public entering the restricted area and using water from a well completed in a aquifer that might be contaminated by releases from the disposal facility (the offsite well water user scenario) reaches a maximum value of 20 percent of the offsite stream scenario, but only after 300 years after facility closure. The probability of an onsite intruder continues to grow, but only reaches 0.5 at the end of the 1,000 year period illustrated in Fig. 2.
IMPACTS ON DOSE PROJECTIONS
The impacts on dose projections of using a probabilistic approach, or conversely waste acceptance criteria expressed in terms such as maximum permissible concentrations of radionuclides in the waste, depend on the half-lives and mobilities of the nuclides. There is little or no effect for short-lived or highly mobile nuclides because they will probably decay to innocuous levels or move well beyond the boundaries of the area under consideration before the end of 100 years of administrative control, when the probabilistic approach begins to make a difference.
With long-lived nuclides, the probabilistic approach can reduce the projected doses to intruders, depending on the duration of effectiveness assumed for intruder barriers, the probabilities assumed for the those barriers, and the final probabilities assumed for onsite food production and using onsite well water. For the assumptions used here that reduction is from a probability of one to a probability of 0.02, a factor of fifty. Even if higher probabilities were assigned to those actions, the reduction in dose could be significant.
The effects of using a probabilistic approach on projected doses from mobile long-lived nuclides is harder to quantify, but can also be significant. Fig. 3 shows the projected offsite well water doses from C-14 at a representative facility with and without the probabilities described above. The representative facility site has a fairly high retardation for carbon. The effect of assigning those probabilities is a reduction of about 80 percent in the peak dose. An almost identical effect was observed for Np-237.
In Fig. 3 the projected annual dose from C-14 when probabilities are not used reaches a peak value a little before 200 years after facility closure. Fig. 2 shows that at 200 years the probability for this scenario is only 0.1 and still rising because the probability of failure of passive institutional controls doesn't reach one until 300 years. The major reduction in the peak projected annual dose, however, results from a probability of using contaminated well water to of only 0.2.
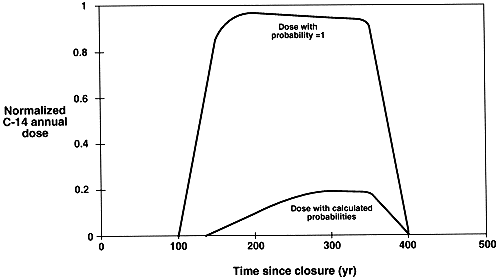
Figure 3. Normalized C-14 Offsite Well Water Doses With and Without Probabilities.
CONCLUSIONS
Probabilities such as those illustrated in the section on calculating probabilities of exposure could be used in calculating probable doses for the purpose of comparing those doses to regulatory dose standards, if those comparisons are permissible. The effect of using probabilities on the projected ability of a specific disposal facility to meet regulatory requirements, or the ability of that facility to receive wastes with different waste forms or wastes with different concentrations of radionuclides, will depend on the details of the pathways to intruding members of the public at that site.
There may never be complete agreement about what probabilities are appropriate at a particular disposal facility site. However, the probabilistic approach to calculating intruder doses appears to be worth considering in future analyses where such doses seem to be an important consideration.
REFERENCES
BACK